This line is supposed to be reaching the end of the universe on both sides

-9-

In the equation (Viii) I have taken into consideration only those push rays which are passing through a single point N and are coming between the lines NM and NK. We can see from the figure that these are not the only rays effecting the two objects B and C. All other rays traveling between between the lines ST and UV are also responsible for the visible attraction between the two objects B and C. Now I will work out the effect of other set of push rays which are traveling between the lines St and UV. Now consider the figure given below. I have extended the the lines ST and UV of previous figure.

The lines ST and UV are crossing at N'. Suppose C' is the another position of object C. Her C and C' are serving as similar filters for all the push rays passing through the point N' and traveling between the lines ST and UV only. The rays will produce same effect on the object C as these will produce on C'. By using equation (Viii) the force (f ') between B and C by all the rays which are passing through the point N' can be worked out to be the same as between B and C'

The line X' Y' is passing through the centers of B and C. This line is supposed to be reaching the end of the universe on both sides. N and N' are the only two points on the line X' Y' which are taken into consideration to work out the resultant effect of push rays which are passing through them along with the objects B and C. We can see that the line X' Y' is nothing but a series of points similar to N and N' from where the other set of push rays can pass and effect the two objects B and C. Now, I will work out the effect of all the push rays passing through B and C while crossing from each point on the line X' Y'.

If I rotate the tangent ST anticlockwise over the circumference of object C till it becomes parallel to X'Y', angle 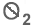will become equal to zero, or the point N' will go to the end of universe towards right hand side. Therefore, the resultant force by all the push rays crossing the line N'Y' and the object B and C will be as under:

Now again, if I rotate tangent LM clockwise over the circumference of object C till it becomes parallel to X'Y', angle will become zero, or point N will go to end of the universe towards left hand side. Therefore, the resultant of all the push rays crossing the line X'N and the object B and C will be as under

Similarly if N is moved to N', angle will become equal to angle . Therefore, the resultant of all the push rays crossing NN' and object B and C will be as under